1. Compressor Impeller Design
1.1. Design Requirements
The design target for the second generation engine is a power output of 10 kw with a fuel-energy-ultilization efficiency, defined as the equation below, of more than 10%.
$$
\eta_\textrm{fuel-exergy-ultilization}=\frac{\text{net power out}}{\text{fuel mass flow rate} \times \text{fuel lower heating value}}=\frac{(1+f)c_p^c T_{t4} \eta_T (1-\Pi^{\frac{1-\gamma^c}{\gamma^c}}) – \frac{c_p^a T_{0}}{\eta_C} (\Pi^{\frac{\gamma^a-1}{\gamma^a}}-1)}{f h_c}
$$
For the consideration of ease of fabrication, the turbine material is selected to be 316 Stainless Steel, which has a yeild strength of more than 120 MPa. For long term exposure to heat, it has less than 0.1% elongation at 1000 h at 85 MPa. Data source: Yagi, K., et al. “Creep properties of heat resistant steels and superalloys.” Landolt Bornstein numerical data & functional relationships in science & technology, Springer, 2nd editions(2004). This decision leads to $T_{t4}=650$ C.
The second generation engine is expected to have a radial compressor and a axial turbine. A conservative estimation of compressor efficiency and turbine efficiecny is made: $\eta_c = 0.8$, $\eta_t = 0.85$.
The fuel is selected to be propane, which has a lower heating value of $h_c = 4.64 \times 10^7$ J / kg.
Some common assumptions are made to the rest of the variables:
- $c_p^a = 1004$ J⋅kg$^{−1}$⋅K$^{−1}$
- $c_p^c = 1200$ J⋅kg$^{−1}$⋅K$^{−1}$
- $\gamma^a = 1.4$
- $\gamma^c = 1.3$
- Compressor Inlet Tempurature $T_0 = 298$ K
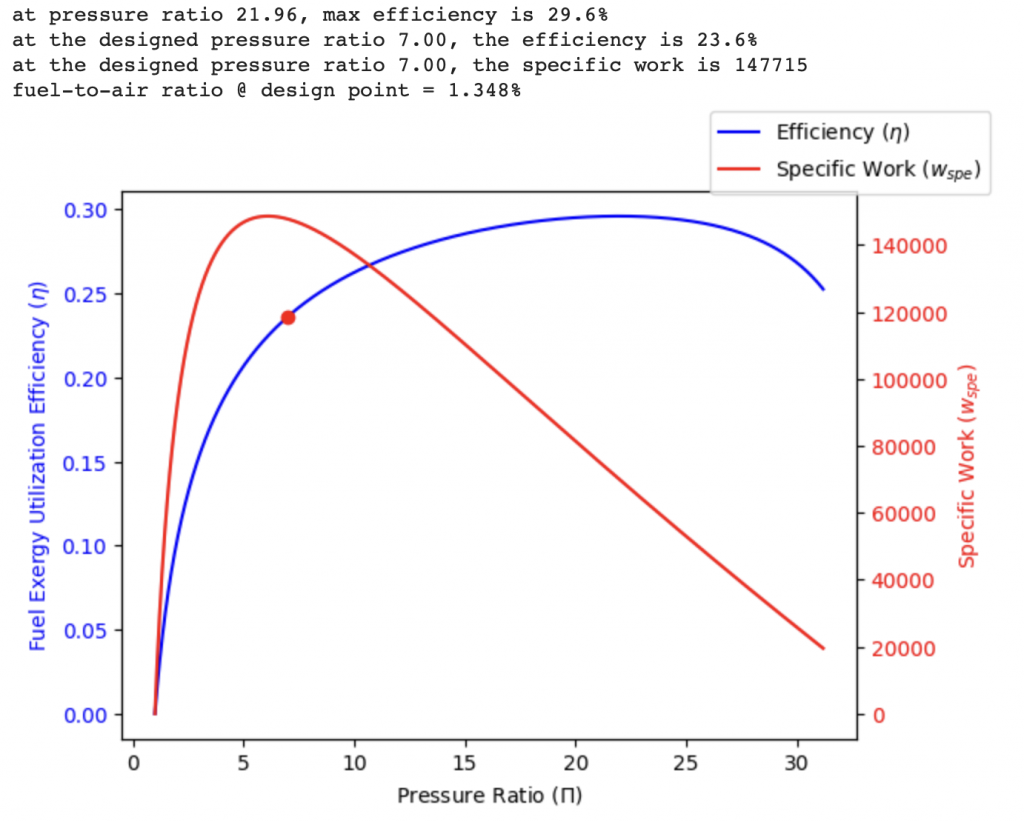
Now, plotting $\eta_\textrm{fuel-exergy-ultilization}$ vs pressure ratio $\Pi$ using this code, we have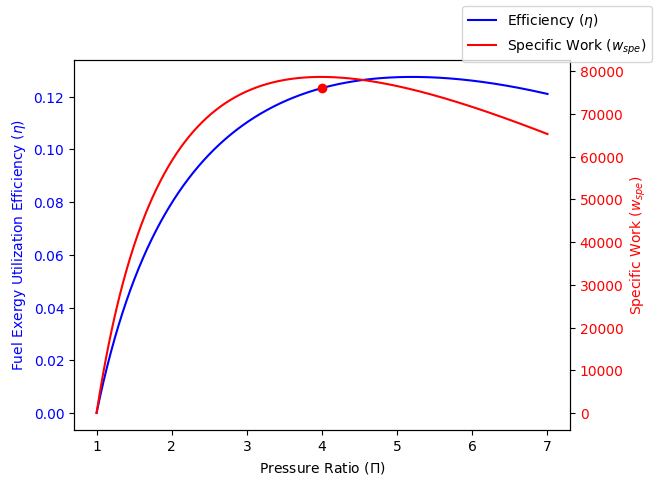 
The pressure ratio is set to 4.0 to maximize specific work. At this design point, specific work is 78473 J/kg and $\eta_\textrm{fuel-exergy-ultilization}$ is predicted to be 13.1%. Fuel-to-air ratio at design point is calculated to be 1.287%.
According to specific work, in order to have 10 kw output, we need a massflow rate of 0.128 kg/s. This is less than half of the massflow rate designed for Gentoo.
According to Kerrebrock, Jack L. Aircraft engines and gas turbines. MIT press, 1992. pp267-268, to maximize efficiency, half of the pressure rise should came from impeller and the other half should cam from diffuser. Therefore, the pressure ratio of the impeller should be 2.0. Which would lead of a blade tip mach number $M_{u2}=1.05$.
Aiming for a compact size, to get to this kind of pressure ratio is almost required to go with hydrid or full ceramic bearings. A very cost effective choice is this hybrid ceramic bearing from GRW, which is rated at 156,000 rpm. Considering Gentoo is design to run at only 60,000 rpm, 156,000 rpm is already a big leap. So, let’s set the upper limit of spool speed to 156,000 rpm.
Due to the limitation of fabrication equippments, we can process rod stock with a diameter up to 200 mm. Say, half of the diameter is researved for diffuser, the impeller diameter $D_w \leq 100$ mm.
As a summary, the following design requirements are selected for the impeller:
- Impeller Pressure Ratio $\Pi_{\text{impeller}} = 2.0$
- Massflow Rate $\dot{m} = 0.128$ kg/s
- Spool Speed $\omega \leq$ 156,000 rpm
- Impeller Diameter $D_2 \leq 100$ mm
1.2. Flow Coefficient
According to Casey, Michael, and Chris Robinson. Radial Flow Turbocompressors. Cambridge University Press, 2021. pp 326, it is common to pick a flow coefficient in the range of $0.07 \leq \phi_{t1} \leq 0.10$. See the figure below (quoted from the book) for the relationship. Therefore a $\phi_{t1} = 0.09$ is selected. 
1.3. Work Coefficien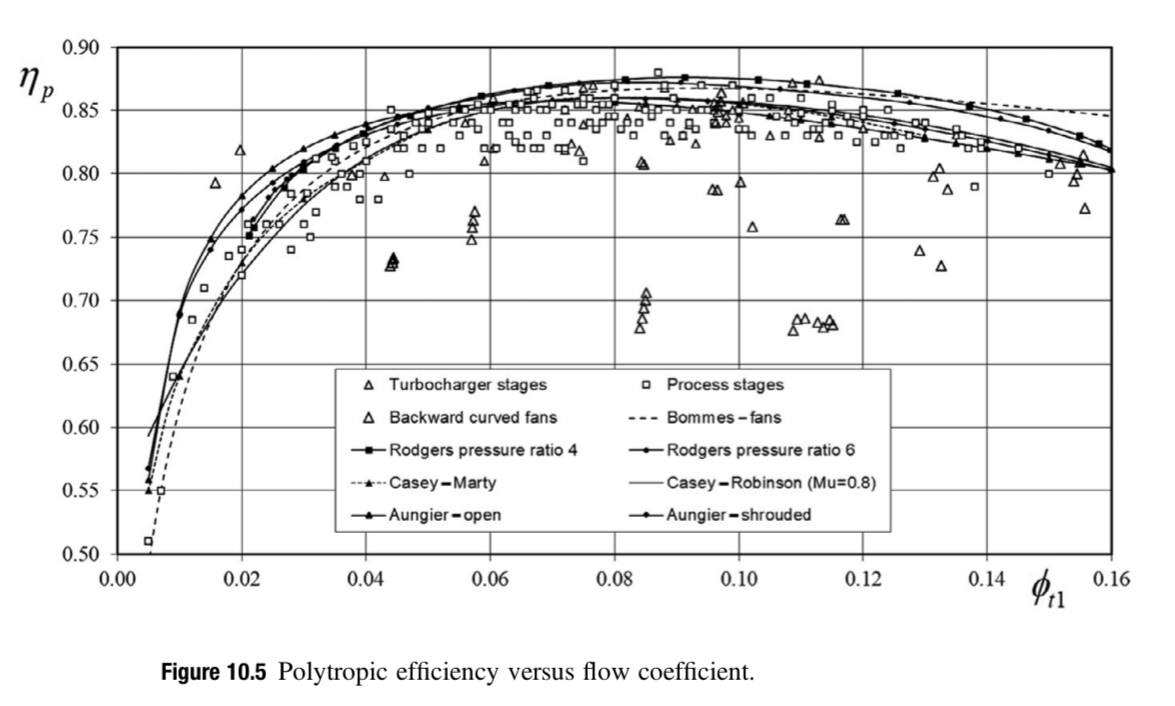t
According to multiple source, it is common to have a work coefficient around 0.7 for higher efficiency and lower tip speed. Therefore, work coefficient is choosen to be $\lambda = \frac{c_{u2}}{u_2} = \frac{\Delta h_t}{(\omega R_2)^2} = \frac{\omega \Delta (rc\theta)}{(\omega R_2)^2} = 0.7$.
1.4. Sizing and Velocities
According to Casey, Michael, and Chris Robinson. Radial Flow Turbocompressors. Cambridge University Press, 2021. pp 330., We have:
$$
M_{u2}^2=\frac{\Pi^{\frac{\gamma-1}{\eta_{\text{ploy}} \cdot \gamma}}-1}{(\gamma-1)\lambda}
$$
In order to get to get an isentropic efficiency of 80% at pressure ratio of 2.0, a polytropic efficiency of 81.9% is required. Therefore, we have $M_{u2}=0.9884$, which matches the prediction result in Kerrebrock, Jack L. Aircraft engines and gas turbines. MIT press, 1992. pp267-268.
The Diameter of the impeller can be then calculated as:
$$
D_2 = \sqrt{\frac{\dot{m}}{\rho_{t1}u_2\phi_{t1}}}
$$
When using $\rho_{t1}=1.293$ kg/m$^3$ and $u_2 = 339$ m/s, we have $D_2$ = 57 mm. The speed is then calculated to be $\omega$ = 113,586 rpm. Both $D_2$ and $\omega$ satisfy our design requirements.
Then the relative Mach number at the inlet tip can be calculated as $M_{w1}=\frac{M_{u2}(\frac{3.2 \phi_{t1}}{k})^{0.36}}{1-0.15M_{u2}(0.45+\frac{\phi_{t1}}{k})} = 0.711$, where impeller inlet shape factor $k = 1-(\frac{r_{1h}}{r_{1c}})^2 = 0.91$ is assumed. Source: Casey, Michael, and Chris Robinson. Radial Flow Turbocompressors. Cambridge University Press, 2021. pp 353.
Then optimal flow inlet angle can be calculated as $\beta_{1c}=cos^{-1}(\frac{\sqrt{3+\gamma M_{w1}^2 + 2 M_{w1}} – \sqrt{3+ \gamma M_{w1}^2-2M_{w1}}}{2M_{w1}})=1.0127 \text{ rad}= 58 ^\circ$. Source: Casey, Michael, and Chris Robinson. Radial Flow Turbocompressors. Cambridge University Press, 2021. pp 348.
The inlet diameter ratio can be then calculated as $\frac{D_{1c}}{D_2}= \frac{M_{w1}\sin (\beta_{1c})}{M_{u2}\sqrt{1+\frac{\gamma -1}{2}M_{w1}^2 \cos ^2{\beta_{1c}}}}=0.6018$ . Source: Rusch, Daniel, and Michael Casey. “The design space boundaries for high flow capacity centrifugal compressors.” Journal of turbomachinery 135.3 (2013): 031035.
Then we know our impeller eye diameter $D_{1c}=34.3$ mm. After that, inlet hub diameter can be calculated using inlet shape diameter $k$, $D_{1h}=D_{1c}\sqrt{1-k}=10.3$ mm.
Impeller relative velocity at the casing can be then calculated as $w_{1c} = u_2 \frac{2D_{1c}}{\sqrt{3}D_2} = 236$ m/s.
A de Haller number of $\text{DH} = 0.6$ is choose for medium diffusion is chosen. Then $w_2= \text{DH} \cdot w_{c1}=142$ m/s.
By ultilizating, outlet velocity triangle, $\frac{w_{u2}-c_s}{c_{m2}}=\tan \beta_2’ $ and the Wiesner correlation $\frac{c_s}{u_2}=\frac{\sqrt{\cos \beta_2’}}{Z^{0.7}}$ where Z is the number of blades at outlet, we can solve for the outlet backsweep angle $\beta_2’=22.0 ^\circ$ if $Z = 10$, or $\beta_2’=24.4 ^\circ$ if $Z = 12$, or $\beta_2’=27.4 ^\circ$ if $Z = 14$. In order to have a high efficiency and side operating range, $Z = 14$ is chosen for a backsweep angle of $\beta_2’=27.4 ^\circ$.
1.5. Velocity Triangles
1.5.1 Inlet Velocity Triangle
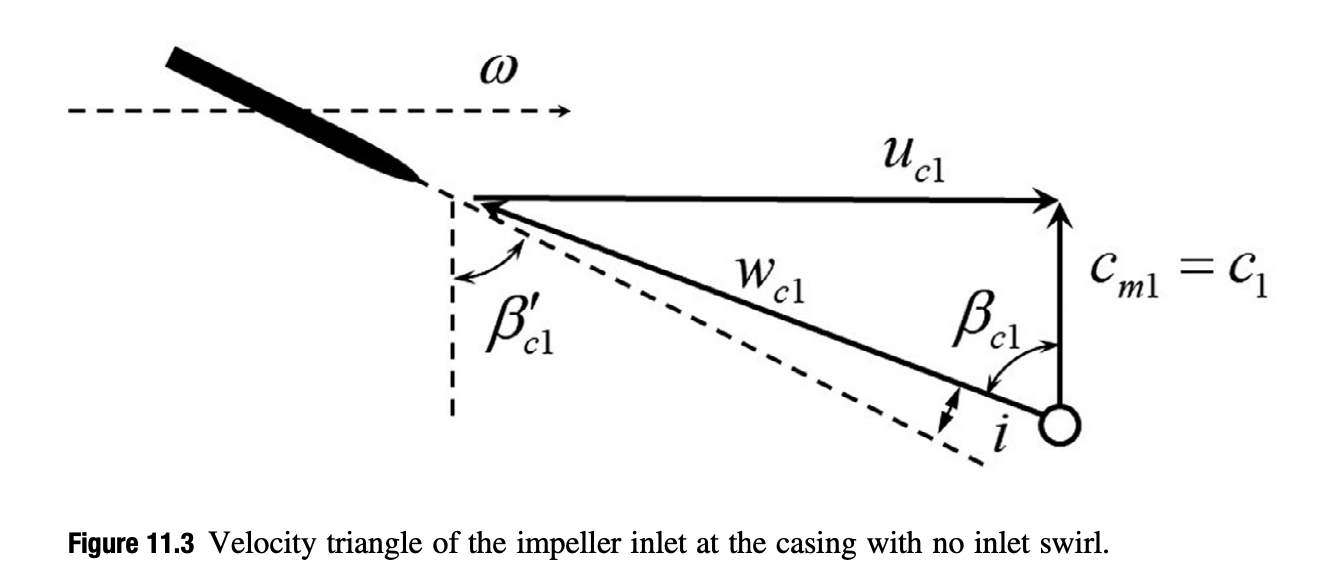
(Figure Source: Casey, Michael, and Chris Robinson. Radial Flow Turbocompressors. Cambridge University Press, 2021.)
- $\omega = $ 113,586 rpm
- $\beta_{c1}=1.0127 \text{ rad}= 58 ^\circ$
- $u_{c1} = 204$ m/s
- $c_{m1}=c_1=119$ m/s
- $w_{c1}=236$ m/s
1.5.2 Outlet Velocity Triangle
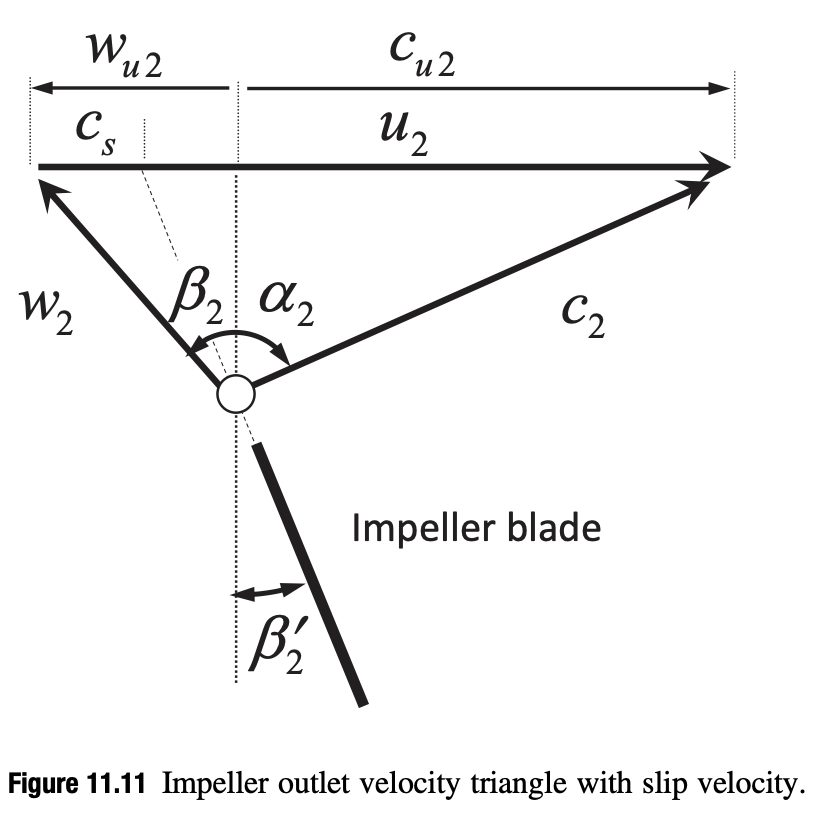
(Figure & Estimation Source: Casey, Michael, and Chris Robinson. Radial Flow Turbocompressors. Cambridge University Press, 2021. See 11.6.6 in this book for outlet velocity triangle design process.)
- $u_2=339$ m/s
- $c_{u2}=\lambda u_2=237.3$ m/s
- $w_{u2}=u_2-c_{u2}=101.7$ m/s
- $w_2 = 142$ m/s
- $c_{m2}=\sqrt{w_2^2-w_{u2}^2}=99.1$ m/s
- $\phi_2=\frac{c_{m2}}{u_2}=$ 0.292
- $c_2 = \sqrt{c_{m2}^2+c_{u2}^2}=257.2$ m/s
- $\alpha_2 = \sin^{-1}(\frac{c_{u2}}{c_2}) = 1.174 \text{ rad} = 67.3 ^\circ$
- $\beta_2 = \sin^{-1}(\frac{w_{u2}}{w_2})=0.798 \text{ rad} = 45.7 ^\circ$
- $\beta_2’= 0.478 \text{ rad} = 27.4 ^\circ$
- $c_s = u_2\frac{\sqrt{\cos \beta_2’}}{Z^{0.7}}=50.4$ m/s
1.6. Verification
1.6.1 Pressure Rise Coefficient
The pressure rise coefficient $\psi=\frac{\Delta h_0}{U^2}=\frac{\Delta p}{\rho \omega^2D_2^2}=\lambda\eta_s=0.56$. According to Casey, Michael, and Chris Robinson. Radial Flow Turbocompressors. Cambridge University Press, 2021. pp 285., an optimal value of $\psi=0.55 \pm 0.05$ is recommended. Our $\psi$ is in the optimal range.
1.6.1 Specific Speed
Then specific speed is calculated as $\omega_s=\frac{\phi^{1/2}}{\psi^{3/4}}=0.463$.
According to Cumpsty, Nicholas A. “Compressor aerodynamics.” Longman Scientific & Technical (1989). pp 20-21., it could offer a good performance despite a bit far away from the optimal value of about $0.7$. See the figure quote from the book for the trend.
2. Turbine Design
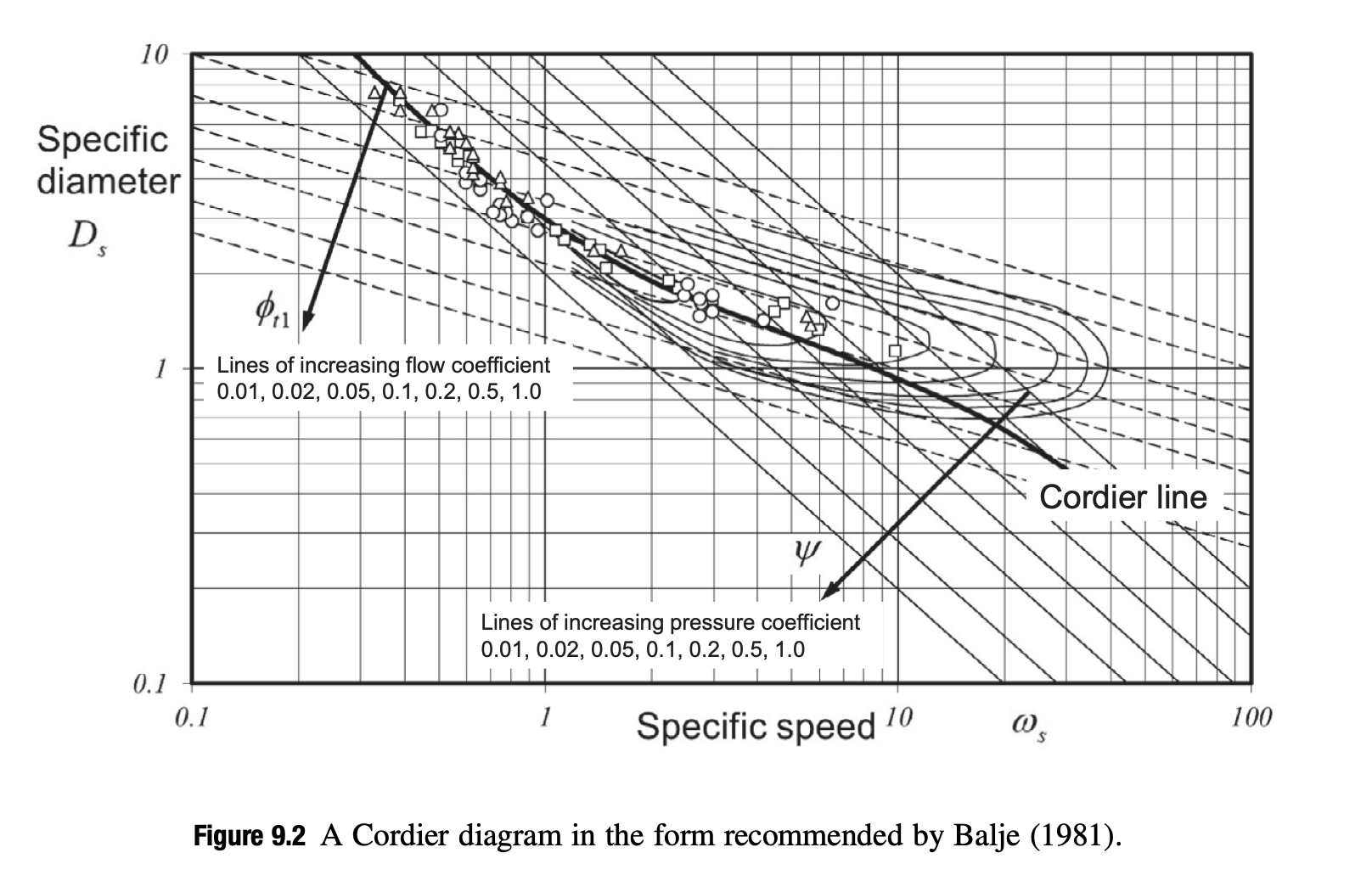
An axial turbine configuration rather than radial turbine is selected for higher efficiency. Since the pressure ratio of compressor is only 4, single stage axial turbine should be able to do the work with fairly high efficiency.
2.1. Flow Coefficient, Work Coefficient, and Degree of Reaction
Multiple versions of Smith Charts are avaliable to help select these parameters. The following values are selected for maximum efficiency:
- Work Coefficient $\Psi = 1.3 = \frac{\Delta h_0}{U^2} = \frac{\Delta c_{\theta}}{U}$
- Flow Coefficient $\phi = 0.6 = \frac{c_x}{U}$
- Degree of Reaction $\text{R} = 0.5 = \frac{h_2-h_3}{h_1-h_3} \approx \frac{p_2-p_3}{p_1-p_3}$
The defination of these parameter can be found at Dixon, S. Larry, and Cesare Hall. Fluid mechanics and thermodynamics of turbomachinery. Butterworth-Heinemann, 2013. pp. 122-123.
A recommanded paper about Smith Chart can be found here: Bertini, Francesco, et al. “A critical numerical review of loss correlation models and Smith diagram for modern low pressure turbine stages.” Turbo Expo: Power for Land, Sea, and Air. Vol. 55232. American Society of Mechanical Engineers, 2013. Here’s one version of Smith Chart from this paper:
2.2. Turbine-Compressor Matching
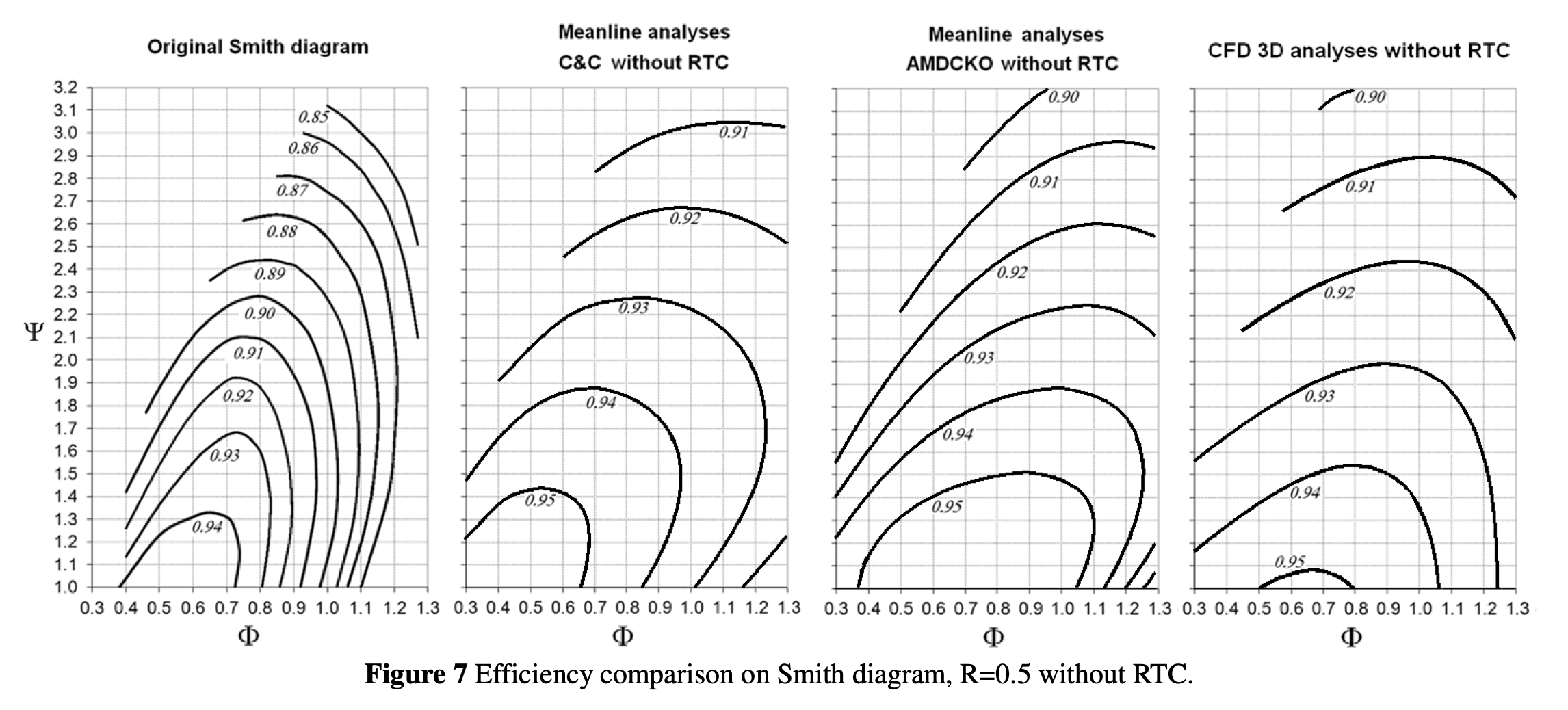
The turbine will be installed on the same shaft with compressor impeller, and therefore $\omega_t = \omega_c = $ 113,586 rpm.
The turbine should be able to supply the right amount of power to run compressor. From the compressor design, the compressor need a specific work of
$$
W_{sp}= \frac{c_p^a T_{0}}{\eta_c} (\Pi^{\frac{\gamma^a-1}{\gamma^a}}-1)= \text{181,757 J/kg}
$$
Assuming there’s a 1% mechanical loss, suggested by Rogers, Gordon Frederick Crichton, et al. Gas turbine theory. Pearson Higher Ed, 2017. pp. 65., the turbine needs to extract $W_{sp}=$ 183,593 J/kg. Therefore, the power needed to run the compressor is $23.5$ kw.
Then the pressure ratio needed for the turbine to provide enough power can be calculated as
$$
\Pi_{\text{turbine}}=(1-\frac{W_{sp}}{(1+f)c_p^c T_{t4} \eta_T })^{\frac{\gamma^c}{1-\gamma^c}} = 2.526
$$
2.3. Blade Speed, Height, and Mean Radius
According to Rogers, Gordon Frederick Crichton, et al. Gas turbine theory. Pearson Higher Ed, 2017. pp. 134., minimal blade tip speed can be calculated as
$$
U \geq \sqrt{\frac{\dot{W}}{\dot{m}\Psi}}=373.4 \text{ m/s}
$$
Using air density $\rho=1.53$ kg/m$^3$ at 4 atm pressure and 650 C, the annulus area can be calculated as
$$
A_x=\frac{\dot{m}}{\rho\phi U}=378.2 \text{ mm}^2
$$
By using the equation
$$
A_x=\pi r_t^2(1-(\frac{r_h}{r_t})^2)
$$
and the following equation from Rogers, Gordon Frederick Crichton, et al. Gas turbine theory. Pearson Higher Ed, 2017. pp. 152. which estimates centrifugal stress
$$
\frac{\sigma_c}{\rho_m}=\frac{(\omega r_t)^2}{2}(1-(\frac{r_h}{r_t})^2)
$$
We can estimates the centrifugal stress (assuming $\rho_m$ = 7980 kg/m$^3$, $\omega=113586 \text{ rpm}=11894.7 \text{ rad/s}$)
$$
\sigma_c = \frac{\rho_m\omega^2A_x}{2\pi}=68 \text{ MPa}
$$
Which is looks good for to satisfty creep resistance requirement.
The blade mean radius can be calculated as $r_m=\frac{U}{\omega}=31.4$ mm, and the blade height can be then calculated as
$$
r_t-r_h=H\approx \frac{\dot{m}}{\rho \phi U 2 \pi r_m}=1.92 \text{ mm}
$$
Therefore, we have turbine hub radius $r_h = 30.4$ mm, and turbine tip radius $r_t=32.4$ mm.
2.4. Velocity Triangles
$\Psi = \frac{\Delta v}{\omega r} = 2\phi \tan \alpha_2-1=2\phi \tan \beta_3-1$, and therefore we have
$$
\alpha_2 = \beta_3 = \tan^{-1}(\frac{\Psi+1}{2\phi})=1.09 \text{ rad}=62.4 ^\circ
$$
and because of $\tan \beta_3 – \tan \beta_2 = \frac{\omega r}{u_2} = \frac{1}{\phi}$
$$
\alpha_3 = \beta_2 = \tan^{-1}(\frac{\Psi-1}{2\phi})=0.245 \text{ rad}=14.0 ^\circ
$$
from previous calculation, we have $\omega r = U = 373.4 \text{ m/s}$ and $c_x=\phi U = 224.0 \text{ m/s}$, and therefore we have
$$
V_{2R} = V_3 = \frac{c_x}{\cos \beta_2} = 230.9 \text{ m/s}
$$
$$
V_2 = V_{3R}=\sqrt{c_x^2 + (c_x\tan \beta_2 + U)^2} = 484.3 \text{ m/s}
$$
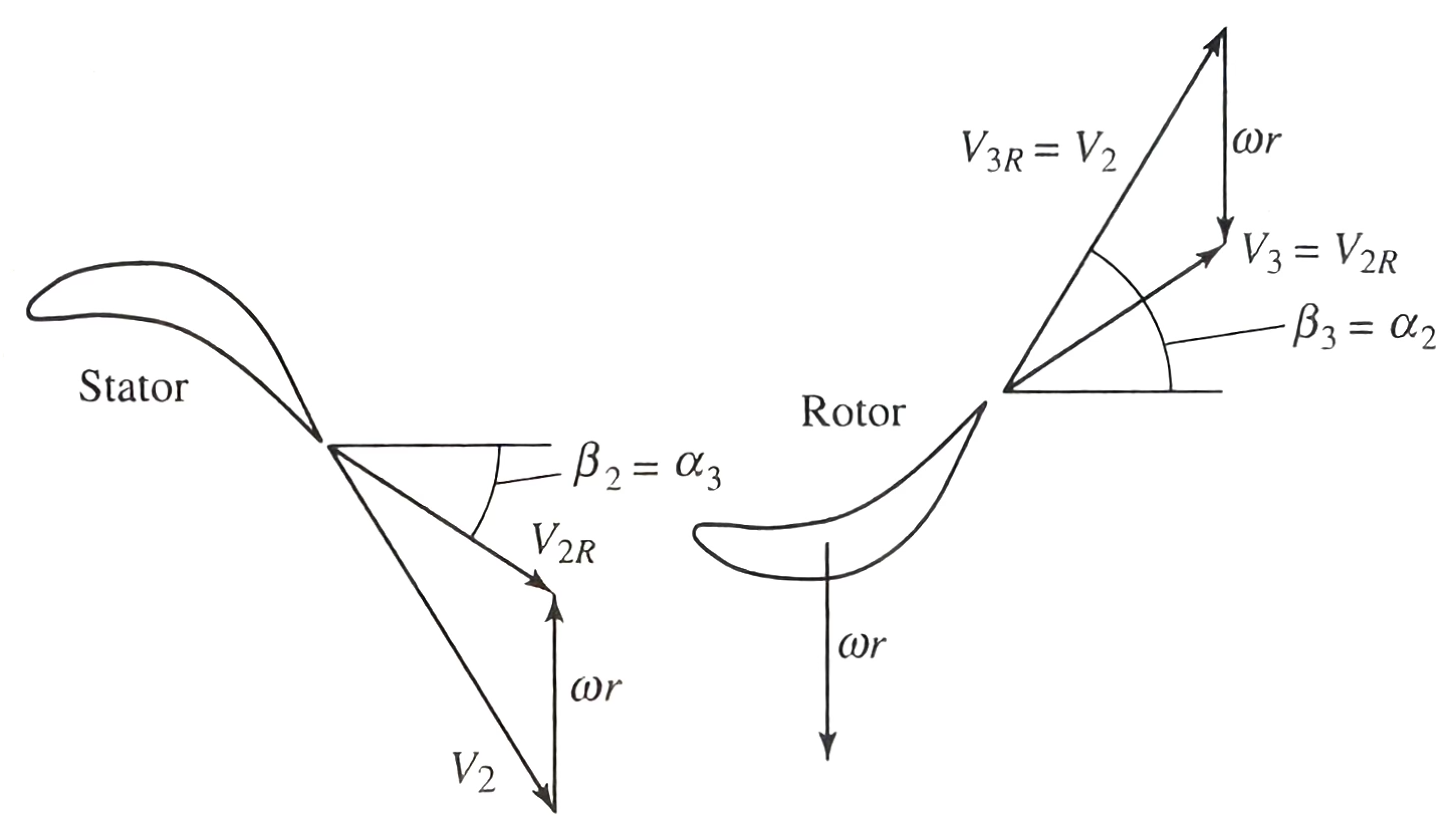
(Figure and Euqation Source: Mattingly, Jack D. Elements of gas turbine propulsion. Vol. 1. New York: McGraw-Hill, 1996. pp. 697.)
